Snellís Law, Reflection, and Refraction
In order to follow the quickest path through a system, a ray changes direction as it travels from a medium of one refractive index to another medium that has a different refractive index.
Snellís Law, which can be stated as
nA Sinθ A = nB Sinθ B
predicts how the ray will change direction as it passes from one medium into another, or as it is reflected from the interface between two media. The angles in this equation are referenced to a surface normal, as is illustrated below.
In the following figure, a ray is incident on an interface between two dissimilar media. A plane that includes the incident ray and a line drawn normal to the surface is called the plane of incidence. This plane also contains the reflected and refracted rays. A refracted ray is transmitted into the second medium and travels in a different direction than the incident ray. The angle that the incident, reflected, and refracted rays make with the surface normal are called the angles of incidence, qi , reflection, qr, and refraction, qt, respectively. The refractive index of medium 1 is n1 and of medium 2 is n2.
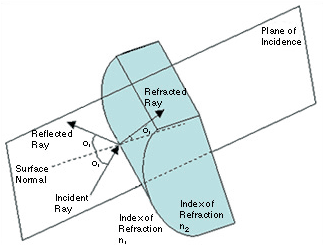
Illustration of incident, reflected, and refracted rays.
In the case of a reflected ray, nA = nB = n2 = n1,
n1 Sinθ i = n1 Sinθ r, which is the same as Sinθ i = Sinθ r.
From this, it is easy to see that the angle of incidence and the angle of reflection are the same!
In the case of the transmitted, or refracted, ray,
n1 Sinθ i = n2 Sinθ t.
If n1<n2, then the angle of refraction is always smaller than the angle of incidence. If n1>n2, then the angle of refraction is larger than the angle of incidenceÖwhen there is an angle of refraction! Imagine the angle of incidence getting larger and larger for the case of n1>n2. Eventually the refracted ray will make an angle of 90° with the surface normal. If the angle of incidence is increased beyond that angle, then refraction does not occur! All of the light incident on the interface is reflected back into the incident medium! The smallest angle of incidence at which total internal reflection occurs is called the critical angle, qc. Using Snellís law,
n1 Sinqθ i = n2 Sin(90°) = n2.
From this,
qc = Sin-1 (n2/n1).

These diagrams illustrate two different cases of refraction. Total internal refraction is depicted in the sketch on the right.
Many devices take advantage of the total internal reflection, including optical waveguides (like optical fiber). A waveguide is a length of transparent material that is surrounded by material that has a lower index of refraction. Rays that intersect the interface between the waveguide material and the surrounding material at angles equal to or larger than the critical angle are trapped in the waveguide and travel losslessly along it.
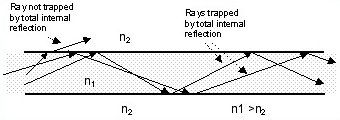
Rays can be trapped in a waveguide through total internal reflection.









