The Refraction of Light
Changing the Speed of Light
Ever notice how your leg looks bent as you dangle it in the water from the edge of a pool? Why do fish seem to radically change position as we look at them from different viewpoints in an aquarium? What makes diamonds sparkle so much?

These are all questions that can be addressed with the important concept of refraction, the bending of light as it encounters a medium different than the medium through which it has been traveling. This meeting place of two different media is called the interface between the media. All refraction of light (and reflection) occurs at the interface.
What happens at the interface to make light refract or reflect? When light is incident at a transparent surface, the transmitted component of the light (that which goes through the interface) changes direction at the interface. Another component of the light is reflected at the surface. As shown in Figure 1, the refracted beam changes direction at the interface and deviates from a straight continuation of the incident light ray.
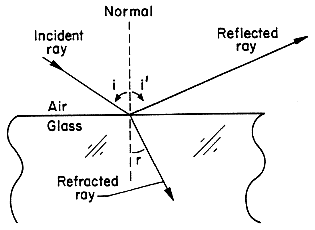
Figure 1. Light in air incident on glass surface where it is partly reflected at the interface and partly transmitted into the glass. The direction of the transmitted ray is changed at the air/glass surface. The angle of refraction r is less than the angle of incidence i.
The change of direction of light as it passes from one medium to another is associated with a change in velocity and wavelength. The energy of the light is unchanged as it passes from one media to another. When visible light in air enters a medium such as glass, the velocity of light decreases to 75% of its velocity in air and in other materials the decrease can be even more substantial. For example, in linseed oil, the velocity decreases to 66% of its velocity in air. Figure 2 displays in bar chart format the velocity of light in different media. The 100% value is the velocity of light in vacuum. For air, the velocity is 99.7% of the speed in vacuum. For some pigments such as titanium (Ti) white, the velocity decreases to 40%.
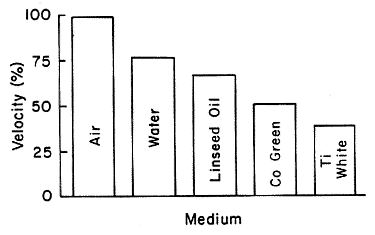
Figure 2. Bar chart of the velocity of visible light in different media. The value of 100% refers to the velocity of light in vacuum.
Waves
Refraction is an effect that occurs when a light wave, incident at an angle away from the normal, passes a boundary from one medium into another in which there is a change in velocity of the light. Light is refracted when it crosses the interface from air into glass in which it moves more slowly. Since the light speed changes at the interface, the wavelength of the light must change, too. The wavelength decreases as the light enters the medium and the light wave changes direction. We illustrate this concept in Figure 3 by representing incident light as parallel waves with a uniform wavelength λ. As the light enters the glass the wavelength changes to a smaller value λ'. Wave "a" passes the air/glass interface and slows down before b, c, or d arrive at the interface. The break in the wave-front intersecting the interface occurs when waves "a" and "b" have entered the glass, slowed down and changed direction. At the next wave-front in the glass, all four waves are now traveling with the same velocity and wavelength λ.
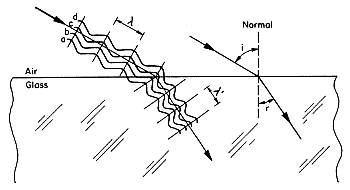
Figure 3. Light waves of wavelength λ incident on glass change direction and wavelength when transmitted into the glass.
The waves are continuous and remain connected as they pass from one medium to another. We can think of it like a long line of people running into the ocean. As the first few people run into the water, they're slowed down because it's harder to run in water. Thus, they bunch up and stay bunched up as they run through the water. When everyone in the line has entered the water, we would see a line of people all running in the same direction, but the line would be shorter and the people would be bunched close together. If they run back to the beach, the first few people would clear the water and run faster. Eventually everyone will have cleared the water and would be running at the original pace with the original spacing between persons.
In this analogy we can think of the whole line of people as the "light wave" and the people themselves as the "crests" of the wave. The distance from one person to her neighbor would be the wavelength of the wave and the water would be the medium into which the light wave is traveling. Why, then does the light wave change direction when it enters the new medium?
We can extend our analogy and imagine two lines of people running into the ocean from the beach. The lines are close together and each person in a line is matched up with another person in the other line. This is analogous to waves a, b, c, and d in Fig. 3 above. When line "a" hits the water first, the line slows down. In order to maintain the one-to-one relationship with the other line, both lines must turn when they hit the water. Which way do they turn? Towards the normal - the imaginary line that runs perpendicular to the interface between the two media (the water and the beach); a pier is a good example of something normal to the water/beach interface.
So the two lines must turn towards the normal when they hit the water. The greater the change in velocity and wavelength, the greater the change in direction. Figure 4 shows the change in direction for light in air incident at 45° on water with refracted angle of 32° and on titanium white (a paint pigment) with a refracted angle of 16°. These angles correspond to the differences in velocity shown in Fig. 2.
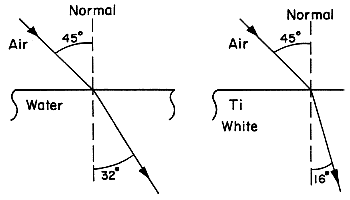
Figure 4. Light incident at 45° on water and Ti white. The angles of refraction (32° for water, 16° for Ti white) depend on the optical properties. The reflected components are not shown.
We can characterize the change in velocity by a number called the refractive index of the material.
The Refraction Index
The ratio of the velocity of light in vacuum to the velocity of light in a medium is referred to as the medium's refractive index, denoted by the letter n. The velocity of light in a vacuum is 3.0 x 108 m/s or about 186,000 miles/s. If we go back to our beach/ocean analogy we can think of the refractive index of the water as something like its density. Air is not very dense at all (its refractive index is 1.0003), so the people run through quite easily; but when they run into water, which is denser than air (and has a refractive index of 1.333), the people slow down and their line bends at the ocean/beach interface. For light, the index of refraction n equals the ratio of the velocities of light in vacuum (c) to that in the medium (v), that is n = c/v.
Refractive indices are most easily determined from the measured values of the incident angle and the angle of refraction and their geometric relationship. Values of the refractive indices for the media shown in Fig. 2 are given below.
| Medium | Refractive Index |
|---|---|
| Air | 1.0003 |
| Water | 1.33 |
| Linseed Oil | 1.48 |
| Co Green | 2.00 |
| Diamond | 2.42 |
| Ti White | 2.5 |
The path of light in air incident on and transmitted through a glass plate is shown in Figure 5. The angle of the incident ray to the normal is 45° and equals that of the reflected ray. The transmitted ray is refracted at an angle of 28° to the normal and exits the glass at an angle of 45° to the normal, an angle equal to that of the incident ray. This explains why, for example, the image we see through a flat-glass window pane is unchanged from that seen through an open window.

Figure 5. Light incident on a glass plate. The reflected part of the ray is shown along with the light path for the refracted component.
Light incident normal to a glass plate does not change direction as the transmitted light continues normal to the surface (air/glass interface). The light is not refracted (that is, no change in angle) but the wavelength and velocity do change. Light does reflect as it encounters the air/glass interface (about 4% in this case).
The paths of light traversing different media are reversible. The same relations are obeyed in Fig. 5, for example, if the light were incident on the bottom of the glass plate. Similarly, in Fig. 4, if the light started in the water, it would be refracted at the water/air interface and would retrace the same reversible path as for light incident from air.
Total Internal Reflection
If light is inside a material such as glass with a larger refractive index n2 than that n1 of the material outside such as air, there is an angle, the critical angle of incidence, beyond which the light is reflected back into the material and does not escape. This is total internal reflection. The critical angle ic is given by
sin ic = n1 / n2
For light exiting glass, n2 = 1.5, the relation becomes
sin ic = 1.0 / 1.5
and the value of the critical angle of incidence is 41°. Light in glass at any angle of incidence to the normal 41° or greater will be reflected from the glass-air interface back into the glass. The bluer area in Fig. 6 is the angular region where light is reflected back into the glass. The greater difference in the two indices of refraction, the smaller the amount of light can escape.
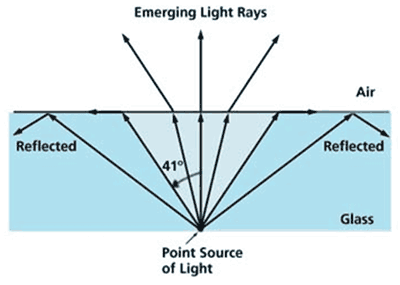
Figure 6. The internal reflectance at an air/glass interface for light rays from a point source in glass. Light rays incident at angles to normal at greater than the critical angle (here, 41° for glass to air) do not leave the material and are reflected at the glass/air interface.
Light fibers and diamonds are both materials with a high refractive index and are used for their properties of "retaining" light.
Chromatic Aberration
Fortunately, the index of refraction of most materials is not the same for each wavelength passing through it; otherwise, we wouldn't see rainbows or the sun's green flash at sunset. When white light passes through a lens or a prism, blue light is bent more than red light. When seen through a lens, this change of index of refraction with wavelength is called chromatic aberration, the change in focal length for different wavelengths of light.
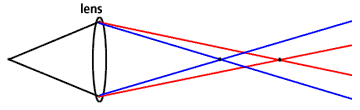
Figure 7. Chromatic aberration in a lens. Blue light is bent more than red light so its focal length is shorter than that of red light, and the "blue" image is located in front of the "red" image.
In precision lens systems, chromatic aberration is undesirable, so achromatic lenses are used to reduce or eliminate this effect. The simplest achromatic lens system is just two lenses with different indices of refraction put together: one convex, one concave. Quality telescopes, microscopes, and camera lenses all have achromatic lens elements.
Want to try activities highlighting these principles? Click on the name of this activity to find an easy, hands-on experiment:









